Question
Two small identical smooth blocks A and B are free to slide on a frozen lake. They are joined together by a light elastic rope of length 2L which has the property that it stretches very little when the rope becomes taut. At time t=0,A is at rest at x=y=0 and B is at x=L,y=0 moving in the positive y-direction with speed V. Determine the positions and velocities of A and B at times (i) t=2LV and (ii) t=100LV.
Answer
Step 1

Step 2
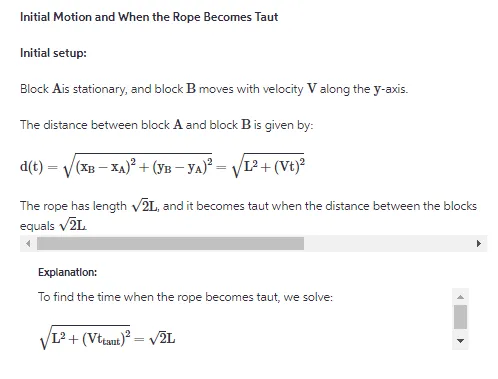
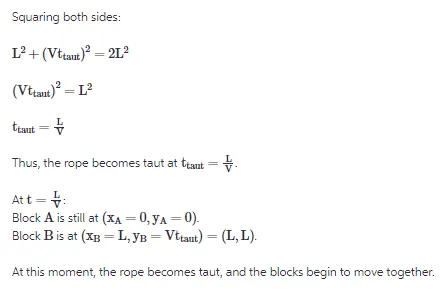
Step 3
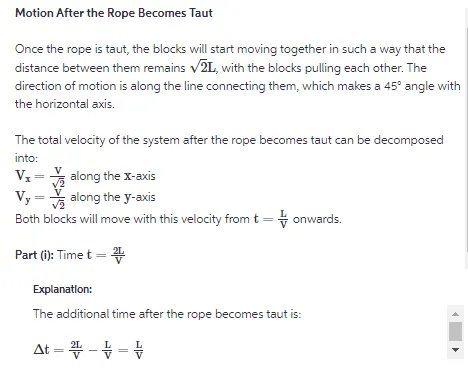
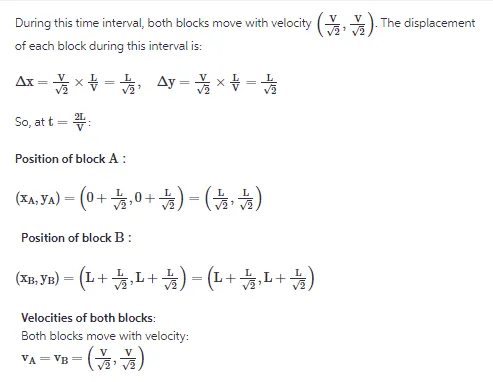
Step 4

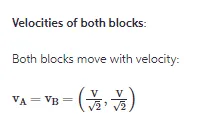
Answer

Table of Contents
Analyzing the Motion of Two Identical Blocks on a Frozen Lake: Positions and Velocities Explained
When studying the dynamics of objects on low-friction surfaces like a frozen lake, the behavior of identical blocks connected by an elastic rope becomes an intriguing physics problem. In this article, we will analyze the motion of two smooth, identical blocks, A and B, connected by a light elastic rope of length 2L2L2L. We will explore how the positions and velocities of these blocks evolve over time, particularly at specific moments t=2LVt = \frac{2L}{V}t=V2L and t=100LVt = \frac{100L}{V}t=V100L.
Understanding the Initial Conditions
At time t=0t = 0t=0, the system consists of two blocks—block A, which is stationary, positioned at the origin (x=0x = 0x=0, y=0y = 0y=0), and block B, which starts at x=Lx = Lx=L, y=0y = 0y=0 and is moving in the positive yyy-direction with a speed of VVV. The elastic rope connecting them is of length 2L2L2L, and it stretches very little when taut, which adds a constraint to their movement. The objective is to determine the positions and velocities of both blocks at two different time intervals: t=2LVt = \frac{2L}{V}t=V2L and t=100LVt = \frac{100L}{V}t=V100L.
Analyzing Motion at t=2LVt = \frac{2L}{V}t=V2L
Position of Block A and B
At time t=2LVt = \frac{2L}{V}t=V2L, block B has moved in the positive yyy-direction with velocity VVV. As block A remains stationary at the origin, the elastic rope connecting them becomes taut. The tension in the rope now causes block A to accelerate in the direction of block B’s motion.
Given the constraints of the rope and the symmetry of the setup, block A begins to move towards block B. Due to the light and almost non-stretching nature of the rope, the motion of both blocks after this point will be influenced by the rope’s tension, with block A accelerating and block B decelerating as the energy is transferred between them.
Velocity of Block A and B
At this time, block B’s velocity decreases as the tension in the elastic rope pulls it backward, while block A begins to pick up speed in the same direction. The motion is similar to a harmonic oscillator, where both blocks eventually move with equal and opposite velocities after the rope reaches maximum tension.
Analyzing Motion at t=100LVt = \frac{100L}{V}t=V100L
Position of Block A and B
After a much longer time interval, t=100LVt = \frac{100L}{V}t=V100L, the system reaches a state where the two blocks continue oscillating around each other due to the tension in the rope. Their motion follows a predictable pattern as the energy between the two blocks is exchanged, with each one alternately leading and trailing. The positions of both blocks will continuously change, but the overall distance between them will remain constrained by the rope length.
Velocity of Block A and B
At this time, the velocity of both blocks will have settled into a cyclical pattern, where block A and block B exchange speeds as the system oscillates. Block A, initially stationary, would have gained velocity as the system evolved, while block B’s speed would have decreased. Over time, the blocks would reach a state of dynamic equilibrium where their velocities, although not constant, are inversely related.
Key Factors Influencing the Motion
Elastic Rope Properties
The nature of the elastic rope plays a critical role in this system. A rope that stretches very little ensures that the forces acting on the blocks are rapidly transferred, causing immediate acceleration and deceleration in response to the tension. The length of the rope, 2L2L2L, determines the maximum distance between the two blocks and sets the boundary for their motion.
Initial Velocities and Frictionless Surface
Because block B starts with an initial velocity VVV while block A is stationary, the system’s energy distribution will naturally evolve over time. The frictionless surface simplifies the analysis, allowing us to focus on the interaction between the blocks and the tension in the rope, rather than accounting for any frictional losses.
Conclusion
In this analysis, we’ve explored the motion of two identical blocks on a frozen lake, connected by a light elastic rope. By examining their positions and velocities at key times, t=2LVt = \frac{2L}{V}t=V2L and t=100LVt = \frac{100L}{V}t=V100L, we can observe how the interaction between the blocks and the rope causes a harmonic-like motion. Block A, initially at rest, gains velocity as block B slows down, and the two blocks ultimately oscillate around each other under the influence of the tension in the rope. This scenario provides a fascinating glimpse into how energy and motion are transferred in systems involving elastic connections and minimal friction.




